BioMath: Exponential Functions

The Biology Project > Biomath > Exponential Functions > Graphing Part II

Exponential Functions

Graphing Part II: Graphs of Transformed Exponential Functions

Nature sometimes gives us problems that cannot be modeled using the basic exponential,

f(x) = a^x.

Most look a bit more complicated, such as this function that we will see when we discuss Newton's Law of Cooling:

T (t) = T_e + (T₀ − T_e ) e^{− kt}

This example illustrates how we often need to transform the most basic exponential function to suit the needs of a specific problem. These graphical transformations include stretches, shrinks, and reflections.

Consider the following mathematical problem:

How would you graph the function g (x) = Ca^x, where C > 0?
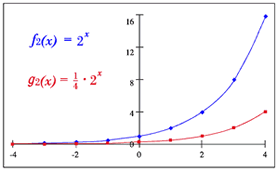
To answer this, think about how g (x) differs from the base function f (x) = a ^x. Recall from the TRANSFORMATIONS SECTION that the constant C > 0 vertically stretches or shrinks the graph of f (x).

The figures below show both a vertical stretch and shrink.

Figure 1	Figure 2
Vertical Stretch , that has been stretched vertically with respect to the base function,	Vertical Shrink , that has been compressed vertically with respect to the base function, f₂ (x) = 2 ^x.
Common Features of Vertically Transformed Functions Notice that the y-intercept in each of these examples has moved from (0,1) for f (x) = a^x to (0,C) for g (x) = Ca^x.

Go to A Shodor activity with the capability of plotting data points as well as a function. You can tweak the function to fit the data.

Now let's examine another type of transformation- reflection across the x-axis.

What does the graph of the function h (x) = e ^-x look like?

Again, ask yourself, "How does the function h (x) compare to the base function
f (x) = e ^x ?". Recall from the graphical transformations section that the negative sign attached to the x indicates a reflection across the y-axis. Therefore, the graph of h (x) = e ^-x should look exactly like the graph of f (x) = e ^x, reflected across the y-axis.

Examine the graphs below.

Notice that the negative sign changes the overall behavior of the graph to the opposite behavior. If you imagine flipping the graph in Figure 3 across the y-axis, the resulting image would look like Figure 4; likewise, if you flip Figure 4 across the y-axis, the resulting image would look like Figure 3.

In this case, the function f (x) = a^x represents exponential growth
(i.e. a > 1),

and its reflection, the function g (x) = f (-x) = a^-x represents
exponential decay.

This makes perfect sense in light of the exponential property (see Laws of Exponents for a review):

Other transformations of exponential functions are also possible, and you should treat them as you would treat transformations of polynomial functions.

*****

In the next section we will discuss the implementation of exponential functions as models for various phenomena.

Look Toward Applications

The Biology Project > Biomath > Exponential Functions > Graphing Part II

The Biology Project
Department of Biochemistry and Molecular Biophysics
The University of Arizona
December 2005
Contact the Development Team